The source is here.

Points of interest

application of the Coulomb and Helmholtz Green's functions
smoothing of the potential and initial guess
manual evaluation of the solution along a line

Background

The Hartree-Fock wave function is computed for the helium atom in three dimensions without using spherical symmetry.

The atomic orbital is an eigenfunction of the Fock operator

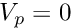
$\begin{eqnarray*} \hat{F} \phi(r) &=& \epsilon \phi(r) \\ \hat{F} &=& -\frac{1}{2} \nabla^2 - \frac{2}{r} + u(r) \\ u(r) &=& \int \frac{\rho(s)}{| r - s |} d^3s \\ \rho(r) &=& \phi(r)^2 \end{eqnarray*}$

that depends upon the orbital via the Coulomb potential ( 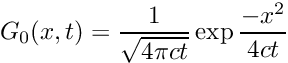) arising from the electronic density ( $\rho(r)$ ).

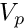
Implementation

Per the usual MADNESS practice, the equation is rearranged into integral form

$\phi = - 2 G_{\mu} * ( V \phi)$

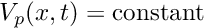
where $\mu = \sqrt{-2E}$ and $G_{\mu}$ is the Green's function for the Helmholtz equation

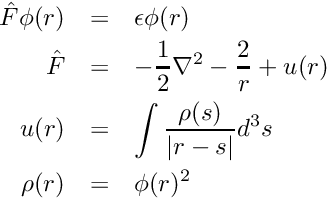
$\left( - \nabla^2 + \mu^2 \right) G(r,r^{\prime}) = \delta(r,r^{\prime})$

The initial guess is 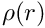$\exp(-2r)$ , which is normalized before use. Each iteration proceeds by

computing the density by squaring the orbital,
computing the Coulomb potential by applying the Coulomb Green's function,
multiplying the orbital by the total potential,
updating the orbital by applying the Helmholtz Green's function,
updating the energy according to a second-order accurate estimate (see the initial MRQC paper), and finally
normalizing the new wave function.

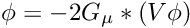
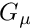
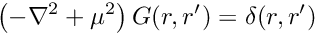
The kinetic energy operator is denoted by $T~=~-\frac{1}{2} \nabla^2$ . Thus, one would expect to compute the kinetic energy with respect to a wave function $\phi$ by $<T \phi, \phi >$ . In this particular example, the wave functions goes to zero smoothly before the boundary so we apply the product rule for differentiation and the kinetic energy is equal to $< \nabla \phi, \nabla \phi >$ .

The source is here.